Multilinear Polynomial Systems: Root Isolation and Bit Complexity
Résumé
We exploit structure in polynomial system solving by considering polyno-mials that are linear in subsets of the variables. We focus on algorithms and their Boolean complexity for computing isolating hyperboxes for all the isolated complex roots of well-constrained, unmixed systems of multilinear polynomials based on resultant methods. We enumerate all expressions of the multihomogeneous (or multigraded) resultant of such systems as a determinant of Sylvester-like matrices, aka generalized Sylvester matrices. We construct these matrices by means of Weyman homological complexes, which generalize the Cayley-Koszul complex. The computation of the determinant of the resultant matrix is the bottleneck for the overall complexity. We exploit the quasi-Toeplitz structure to reduce the problem to efficient matrix-vector multiplication, which corresponds to multivariate polynomial multiplication, by extending the seminal work on Macaulay matrices of Canny, Kaltofen, and Yagati [9] to the multi-homogeneous case. We compute a rational univariate representation of the roots, based on the primitive element method. In the case of 0-dimensional systems we present a Monte Carlo algorithm with probability of success 1 − 1/2^r, for a given r ≥ 1, and bit complexity O_B (n^2 D^(4+e) (n^(N +1) + τ) + n D^(2+e) r (D +r)) for any e> 0, where n is the number of variables, D equals the multilinear Bézout bound, N is the number of variable subsets, and τ is the maximum coefficient bitsize. We present an algorithmic variant to compute the isolated roots of overdetermined and positive-dimensional systems. Thus our algorithms and complexity analysis apply in general with no assumptions on the input.
Fichier principal
 emt-bhomo-jsc.pdf (393.29 Ko)
Télécharger le fichier
emt-bhomo-jsc.pdf (393.29 Ko)
Télécharger le fichier
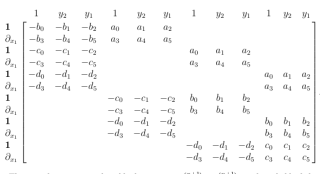 vignette.png (29.97 Ko)
Télécharger le fichier
vignette.png (29.97 Ko)
Télécharger le fichier
| Origine | Fichiers produits par l'(les) auteur(s) |
|---|
| Format | Figure, Image |
|---|
Loading...