Adequate Inner Bound for Geometric Modeling with Compact Field Function
Résumé
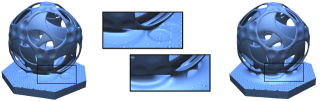
Recent advances in implicit surface modeling now provide highly controllable blending effects. These effects rely on the field functions of $\mathbb{R}^3 \rightarrow \mathbb{R}$ in which the implicit surfaces are defined. In these fields, there is an outside part in which blending is defined and an inside part. The implicit surface is the interface between these two parts. As recent operators often focus on blending, most efforts have been made on the outer part of field functions and little attention has been paid on the inner part. Yet, the inner fields are important as soon as difference and intersection operators are used. This makes its quality as crucial as the quality of the outside. In this paper, we analyze these shortcomings, and deduce new constraints on field functions such that differences and intersections can be seamlessly applied without introducing discontinuities or field distortions. In particular, we show how to adapt state of the art gradient-based union and blending operators to our new constraints. Our approach enables a precise control of the shape of both the inner or outer field boundaries. We also introduce a new set of asymmetric operators tailored for the modeling of fine details while preserving the integrity of the resulting fields.
Fichier principal
 SMI2013_Canezin_et_al.pdf (3.54 Mo)
Télécharger le fichier
SMI2013_Canezin_et_al.pdf (3.54 Mo)
Télécharger le fichier
 complex_object_large.png (841.15 Ko)
Télécharger le fichier
complex_object_large.png (841.15 Ko)
Télécharger le fichier
Origine : Fichiers produits par l'(les) auteur(s)
Format : Figure, Image
Loading...